CDE will be closed Wednesday, Nov. 27 through Friday, Nov. 29 for the Thanksgiving holiday.
You are here
Features and Changes to Look For in the 2020 Mathematics Standards
Standards Menu
Features and Changes to Look For in the 2020 Mathematics Standards
The revision of the Colorado Academic Standards for mathematics brought about a number of noteworthy changes. While none of the changes fundamentally altered the content that students are expected to learn, educators need to be aware of these changes to fully benefit from the revisions to the standards document. These features and changes are organized into six sections:
- A Reorganization that Brings Greater Focus to Connected Ideas
- Promoted Practices and Prepared Graduates
- Inquiry Questions
- Coherence Connections
- Preschool and Early Elementary
- Advanced High School Standards
A Reorganization that Brings Greater Focus to Connected Ideas
The 2010 mathematics standards often brought many evidence outcomes (EOs) together under a single grade level expectation (GLE). EOs were grouped using first-level EOs, multiple second-level EOs, and occasionally third-level EOs, and any level could contain footnotes.
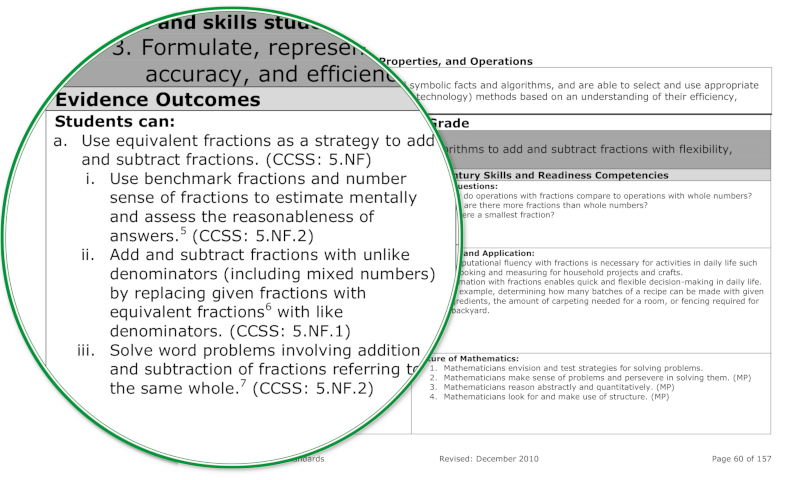
2010 Mathematics Standards

2020 Mathematics Standards
Guided by public feedback, misalignment issues cited in a benchmarking report, and their own experience with the standards, the review and revision committee reorganized the standards into a greater number of GLEs, each more tightly focused on a mathematical idea with fewer EOs.
The committee combined some EOs and integrated any footnotes. In all but a small number of cases, the committee retained the language of individual EOs. These changes were made to add specificity and clarity, not to fundamentally change what students are expected to learn.
As an example, the first GLE under Standard 2 at high school in the 2010 standards had six first-level EOs, a total of 19 second-level EOs, and three third-level EOs. In addition, there were 11 footnotes used in these EOs, each containing an example of the mathematics described by an EO. The footnotes appeared four pages later in the text.
The mathematics review and revision committee reorganized this single 2010 GLE into six GLEs for the 2020 standards, one for each of the first-level EOs. Each first-level EO became a “new” GLE, and the sub-EOs from 2010 became first- and second-level EOs in 2020. The added benefit of these kinds of revisions across the standards is not only a tighter clustering of mathematical ideas, but each “new” GLE is supported by its own set of Academic Context and Connections (previously known as 21st Century Skills and Readiness Competencies).
Below is an annotated example of how larger 2010 GLEs have been reorganized into multiple 2020 GLEs. This type of reorganization is typical of the reorganization found across all grades and standards categories in the 2020 mathematics standards.

A 4th Grade example of the reorganization of GLEs and EOs from 2010 to 2020
Promoted Practices and Prepared Graduates
The 2010 Colorado Academic Standards introduced the Standards for Mathematical Practice under the subheading Nature of Mathematics. Only the titles of the Practices were included, along with a simple “(MP)” citation.
Since 2010, mathematics educators across Colorado have embraced the Practices and elevated their status to support the learning of mathematical content with the strategies and techniques of mathematicians.
The 2020 mathematics standards review and revision committee received pro-Practices feedback from the public and used their own experience to decide that the Practices should take a more prominent position in the revised standards.

2010 Mathematics Standards
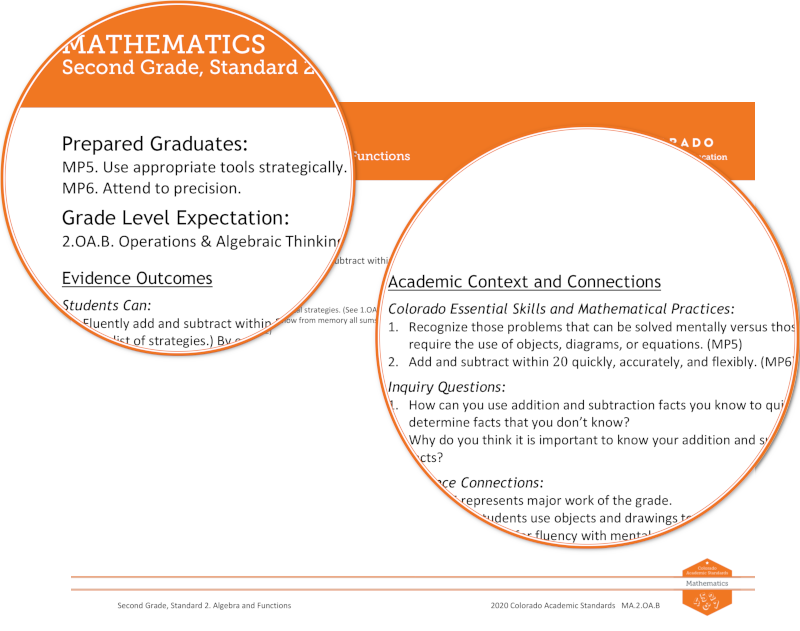
2020 Mathematics Standards
In the 2020 standards, the Standards for Mathematical Practice appear as Prepared Graduate statements. These represent concepts and skills all graduates need to be successful after high school. Although students should have opportunities to engage in all eight Standards for Mathematical Practice when learning any mathematical content, the review and revision committee chose to align GLEs to only those practices for which there was the greatest opportunity to engage in each practice.
In addition, the committee replaced the Nature of Mathematics subheading with Colorado Essential Skills and Mathematical Practices. These are content-specific descriptions of each Practice named as a Prepared Graduate statement.
Inquiry Questions
The review and revision committee kept the Inquiry Questions subheading from 2010 but made significant edits and additions. The committee designed each question to prompt deeper thinking about the mathematical content described by the grade level expectations and evidence outcomes.
Coherence Connections
The review and revision committee replaced the 2010 Relevance and Application subheading with Coherence Connections. This new subsection is designed to help teachers quickly connect mathematical ideas to other content in previous, current, and future grades.
Coherence Connections also indicates whether the mathematics described by a grade level expectation is major, supporting, or additional work of each grade.
In high school, Coherence Connections helps teachers interpret how grade level expectations and evidence outcomes fit within a coherent system of teaching and learning mathematics. This can include explaining the relevance and application of the mathematics, thus retaining some of those ideas from the 2010 standards.

2020 Mathematics Standards
Preschool and Early Elementary
The review and revision committee’s preschool revisions are a significant change compared to the 2010 standards, but a minor adjustment when compared with CDE’s 2013 Early Learning Development Guidelines and the 2015 Head Start Early Learning Outcomes Framework.

2010 Mathematics Standards

2020 Mathematics Standards
In other elementary grades, the review and revision committee shifted some content to fill standards categories that had previously been left empty. This shifted content represents the same content as was in the 2010 standards but is now located under a different category in the same grade. Most content focused on operations is now found under “Algebra and Functions” and measurement-focused content is now found under “Data, Statistics, and Probability.”
Advanced High School Standards
New in the 2020 high school standards are advanced evidence outcomes, designated with a (+) symbol. The review and revision committee included these outcomes to help support students in their last few semesters of high school who wish to prepare for future study in mathematics or other STEM fields.
In making this decision, the committee consulted public feedback from the 2010 standards, advanced standards in the Common Core State Standards, research about the relevance of mathematics for college and careers, guidance used by curriculum publishers, and testing frameworks from SAT and PARCC. For each evidence outcome identified as potentially advanced by public feedback and members of the committee, the committee checked these resources to ensure that any 2020 evidence outcomes marked as advanced (+) earned that designation. Below are those HS evidence outcomes that have been designated as advanced in addition to those already labeled (+) in the CCSS. (HS.A-SSE.B.4 was split into two evidence outcomes, with deriving the formula marked as advanced.)

2020 Mathematics Standards
Standard 1: Number and Quantity
- (+) Explain why the sum or product of two rational numbers is rational; that the sum of a rational number and an irrational number is irrational; and that the product of a nonzero rational number and an irrational number is irrational. (CCSS: HS.N-RN.A.3)
Standard 2: Algebra and Functions
- Use the formula for the sum of a finite geometric series (when the common ratio is not 1) to solve problems. For example, calculate mortgage payments.★ (CCSS: HS.A-SSE.B.4)
- a. (+) Derive the formula for the sum of a finite geometric series (when the common ratio is not 1). (CCSS: HS.A-SSE.B.4)
- (+) Prove polynomial identities and use them to describe numerical relationships. For example, the polynomial identity (x^2+y^2)^2 = (x^2-y^2)^2 + (2xy)^2 can be used to generate Pythagorean triples. (CCSS: HS.A-APR.C.4)
- (+) Use radian measure of an angle as the length of the arc on the unit circle subtended by the angle. (CCSS: HS.F-TF.A.1)
- (+) Explain how the unit circle in the coordinate plane enables the extension of trigonometric functions to all real numbers, interpreted as radian measures of angles traversed counterclockwise around the unit circle. (CCSS: HS.F-TF.A.2)
- (+) Model periodic phenomena with trigonometric functions with specified amplitude, frequency, and midline.★ (CCSS: HS.F-TF.B.5)
- (+) Prove the Pythagorean identity sin^2(θ) + cos^2(θ) = 1 and use it to find sin(θ), cos(θ), or tan(θ) given sin(θ), cos(θ), or tan(θ) and the quadrant of the angle. (CCSS: HS.F-TF.C.8)
Standard 3: Data, Statistics, and Probability
No additional evidence outcomes were identified as advanced.
Standard 4: Geometry
- (+) Construct an equilateral triangle, a square, and a regular hexagon inscribed in a circle. (CCSS: HS.G-CO.D.13)
- (+) Derive using similarity the fact that the length of the arc intercepted by an angle is proportional to the radius, and define the radian measure of the angle as the constant of proportionality; derive the formula for the area of a sector. (CCSS: HS.G-C.B.5)
- (+) Derive the equation of a parabola given a focus and directrix. (CCSS: HS.G-GPE.A.2)



Connect With Us




